Effects of fracture network connectivity in coal seams on proppant transport
-
摘要:
煤层气开发过程中采用的水平井分段多簇压裂会在煤层内与割理共同形成复杂裂缝网络，且由于割理发育和开启程度不同，各簇缝网之间的联通性存在差异，直接影响支撑剂在缝网内的输送。为了探究煤层内各簇缝网的联通情况对支撑剂铺置情况的影响，建立了联通和未联通两种裂缝网络，该裂缝网络由水平井段串联两簇水力裂缝并与正交割理组合，两簇缝网之间的联通情况取决于二级裂缝的联通性。在考虑支撑剂球度的基础上，采用Eulerian-Eulerian的多相流模型中的Mixture模型模拟不同携砂液黏度、携砂液排量和支撑剂粒径条件下联通和未联通裂缝网络中支撑剂在各级裂缝中的分布特征，并对比两种裂缝网络中支撑剂的填砂比例明确裂缝网络联通性对裂缝内支撑剂铺置特征的影响。模拟结果表明，由于联通缝网中分别由两簇裂缝进入的携砂液在缝网联通部分互相干扰，该联通部分几乎没有支撑剂分布。但是与未联通裂缝相比，支撑剂在联通缝网的主裂缝内输送距离更远。就裂缝网络整体的填砂比例而言，未联通缝网的整体填砂比例要远高于联通缝网，在本研究的模拟参数条件下平均高出53.3%。联通和未联通裂缝网络中各级裂缝内铺砂浓度小于5%的区域均占较大比例，其次是铺砂浓度大于10%的区域，铺砂浓度介于5%～10%的区域最小。同时降低携砂液黏度、提高携砂液排量、使用粒径较小的支撑剂均可以有效改善两种缝网中支撑剂的铺置情况，特别是联通缝网中携砂液排量应不小于12 m3/min，支撑剂粒径不大于100 目（0.15 mm）。
Abstract:The horizontal well staged multi-cluster fracturing used in the development of coalbed methane could jointly form a complex fracture network with cleats in the coal seam. Due to the different density and aperture of cleats, there are differences in the connectivity between various clusters of fracture networks, which directly affects the proppants transport within the fracture network. In order to explore the influence of the connectivity of various clusters of fracture networks in coal seams on the placement of proppants, two types of fracture networks, connected and unconnected, were established. The fracture network consists of two clusters of hydraulic fractures connected in series by a horizontal well section and combined with orthogonal cleats. The connectivity between the two clusters of fracture networks depends on the connectivity of secondary fractures. Considering the proppants sphericity, the Mixture model in the multiphase flow model of Eulerian Eulerian is used to simulate the distribution characteristics of proppants in different levels of fractures in connected and unconnected fracture networks under different slurry viscosity, slurry displacements, and proppant particle sizes. The sand filling ratio of proppants in these two types of fracture networks is compared to clarify the influence of network connectivity on the proppants distribution in fractures. The simulation results indicate that due to the interference between the sand carrying fluids entering from two clusters of fractures in the connecting part of the fracture network, there is almost no proppant in this connecting part. However, compared to unconnected fracture networks, the proppant is transported further within the main fracture of the connected fracture network. In terms of the total sand filling ratio of the fracture network, the sand filling ratio of the unconnected fracture network is much higher than that of the connected fracture network, with an average increase of 53.3% under the simulation parameter conditions of this study. In both connected and unconnected fracture networks, areas with sand concentration less than 5% in each level of fracture account for a large proportion, followed by areas with sand concentration greater than 10%, and areas with sand concentration between 5% and 10% have the smallest proportion. Further, reducing the slurry viscosity, increasing the slurry displacement, and using smaller particle size proppants can effectively improve the proppants distribution in both types of fracture networks. Especially, the slurry displacement in the interconnected fracture network should not be less than 12 m3/min, and the particle size of the proppant should not larger than 100 mesh.
-
Keywords:
- coalbed methane /
- hydraulic fracturing /
- fracture network /
- proppant transport /
- mixture model
-
0. 引 言
我国煤层气开发在天然气增储上产方面表现出巨大的潜力，是维护国家能源安全的重要保障[1-2]。水平井分段多簇压裂与煤层中发育的割理系统形成复杂裂缝网络，成为开发该非常规天然气资源的主要技术手段[3-5]，其中支撑剂在裂缝网络中的分布情况直接决定了压后气井的产能。
国内外学者针对煤层压裂过程中支撑剂在裂缝内的运移规律开展了大量研究，其中陈捷等[6]采用数值模拟并结合工程试验，研究了薄煤层中不同宽度裂缝内支撑剂的铺砂面积和有效支撑长度。苏现波等[7]借助支撑剂运移的可视化实验设备，研究了裂缝形态和压裂液性质对支撑剂分布的影响。王生维等[8]先观察了采煤巷道中发现的压裂支撑剂形貌和堆积特征，进而对压裂时支撑剂的沉积过程进行还原。张苗等[9]认为煤层压裂中陶粒的适用性要好于石英砂，并采用物理实验与数值模拟相结合的方法研究了不同粒径配比的陶粒支撑剂的运移规律。LI等[10]采用高压注砂三轴水力压裂实验系统研究了支撑剂的运移分布，发现裂缝入口粗糙度和整体粗糙度分别决定了支撑剂的输送方向和分布形式。LAMEI等[11]将实验和数字岩石技术相结合，研究了将支撑剂注入天然压裂煤样品的方法。
水力裂缝与煤层割理构成了复杂裂缝网络，携砂液在各级裂缝中分流导致支撑剂的分布特征更加复杂。郭天魁等[12]采用CFD-DEM耦合方法，分析了施工排量、支撑剂密度和压裂液黏度对复杂裂缝中支撑剂运移铺置的影响。张艳博等[13]建立了二级裂缝的位置和倾角可变的缝网模型，探究了复杂缝网中二级裂缝形态对支撑剂输送特征的影响。XU等[14]构建了三维迂曲裂缝网络并在考虑携砂液、裂缝表面和支撑剂之间的相互作用的基础上，模拟了迂曲裂缝网络中支撑剂的分布特征。MAO等[15]采用MP-PIC方法直接模拟了支撑剂的压裂施工现场的输送过程，包括携砂液从井筒输送通过射孔孔眼，最终进入裂缝。杨鹏等[16]基于位移不连续方法和欧拉−欧拉法建立了井平台多井压裂模式下三维裂缝扩展与支撑剂运移耦合模型，以实际井平台参数为例研究了多井压裂裂缝扩展形态与缝内支撑剂铺置规律。
上述针对多簇裂缝内支撑剂分布的研究只是利用水平井筒将多簇孤立裂缝串联，多簇裂缝在缝网内部彼此联通开展的模拟研究很少，更缺乏多簇缝网联通性对支撑剂输送影响的分析。为此，本研究将煤层割理与多簇水力裂缝结合，构建联通和不联通的两种缝网形式，利用Eulerian-Eulerian多相流模型中Mixture模型对不同携砂液黏度、排量和支撑剂粒径条件下两种缝网内支撑剂的分布特征开展对比模拟。
1. 煤层中不同联通性缝网模型
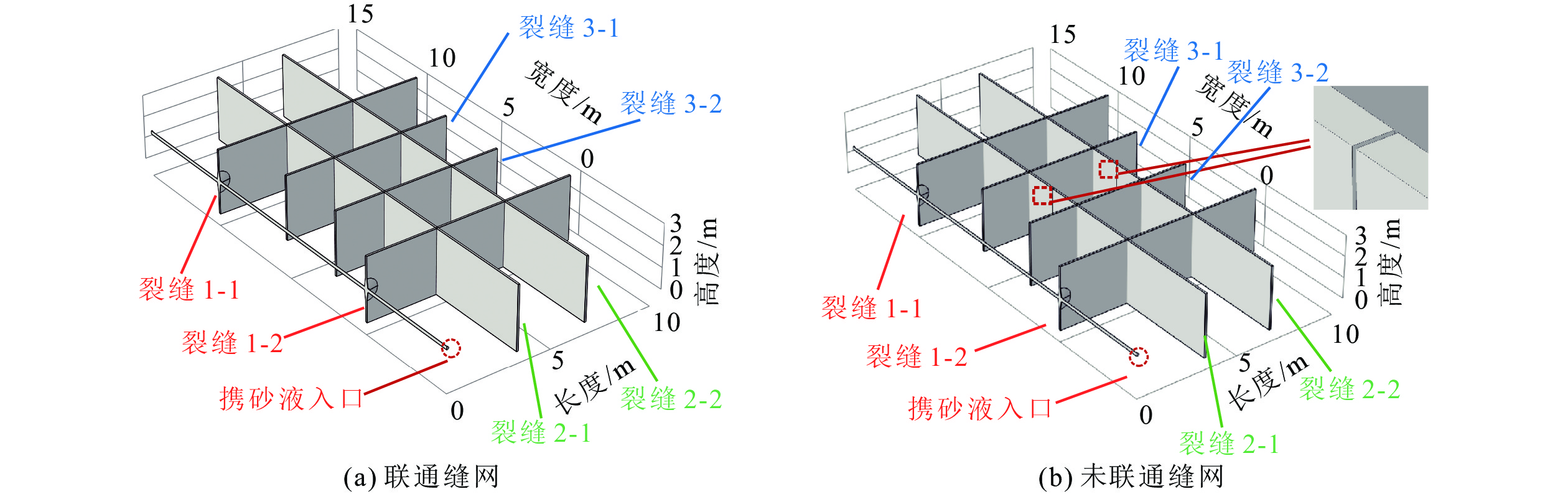
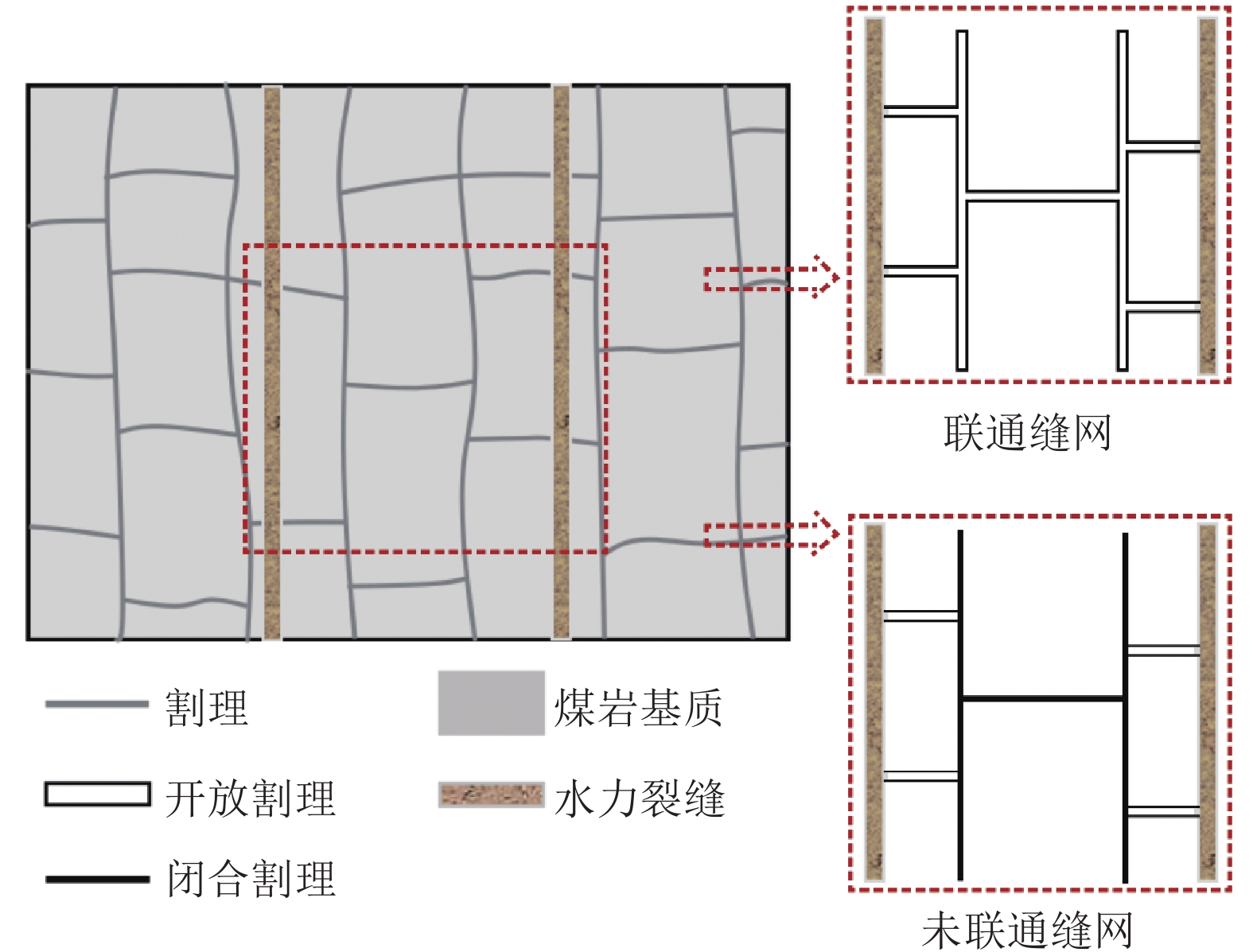
在煤层开展水力压裂之后，即形成了包括煤岩基质、煤层割理和多簇水力裂缝并存的渗流系统，如图1所示。各簇水力裂缝之间发育煤层割理，割理本身可能是开放的，也可能是封闭的，这样就会形成联通缝网和未联通缝网两种形式。
![]()
图 1 煤层水力压裂后复杂缝网示意Figure 1. Schematic diagram of complex fracture networks after hydraulic fracturing in coal seam针对上述两种缝网形式，分别建立了对应的缝网几何模型并以此进行数值模拟，如图2所示。缝网模型包括水平井筒、两条主裂缝（裂缝1-1和裂缝1-2）、两条二级裂缝（裂缝2-1和裂缝2-2）以及两条三级裂缝（裂缝3-1和裂缝3-2）。鉴于煤层割理的正交特性，各级裂缝彼此垂直。两种缝网的区别在于，联通缝网的二级裂缝是贯通的，而未联通缝网的二级裂缝在中间位置断开，断开面作为后续模拟中携砂液出口。两种缝网具体几何参数见表1[17-19]，且由于联通缝网内支撑剂在各簇裂缝间的窜流主要集中在近井筒附近，因此主裂缝长度设置为10 m。
![]()
图 2 数值模拟中缝网几何模型Figure 2. Geometric model of fracture networks in numerical simulation表 1 缝网几何参数Table 1. Geometric parameters of fracture networks参数 数值 主裂缝长度/m 10 三级裂缝长度/m 8 裂缝宽度/cm 1 水平井筒长度/m 20 簇间距/m 10 三级裂缝间距/m 3.33 联通二级裂缝长度/m 20 未联通二级裂缝长度/m 9.995 裂缝高度/m 3 水平井筒半径/cm 7 二级裂缝间距/m 3.33 未联通缝网簇间二级裂缝间距/m 0.01 2. 携砂液输送模型
Eulerian-Eulerian的多相流模型是模拟固体支撑剂颗粒在携砂液中运动分布的重要方法，该方法主要包含VOF模型（流体体积模型）、Eulerian模型（欧拉模型）和Mixture模型（混合模型）。其中，流体体积模型主要用于分层流和自由表面流的求解模拟[20]，欧拉模型求解多相流时，常将动量方程和连续性方程分开求解，计算量较大[21-22]。而混合模型是欧拉模型的一种简化形式，适用于支撑剂在裂缝网络中的输送模拟。同时，混合模型计算量较小且精度高[23]，因此本研究采用此方法对缝网中支撑剂分布情况进行模拟。
在混合模型中，颗粒−流体组合被视为具有密度和黏度等宏观特性的单一流动连续体。两相由分散相和连续相组成，且通常连续相是液体，而分散相可以是固体颗粒、液滴或气泡。混合物模型依赖于以下假设：① 混合物中各相的密度恒定；② 混合物中两相的压力场相同；③ 与宏观流动的时间尺度相比，粒子弛豫时间较短。
使用混合流模型计算裂缝内砂浓度分布，其动量方程[24-25]为
$$ \begin{split} & \rho \frac{{\partial j}}{{\partial t}} + \rho \left( {j \cdot \nabla } \right)j + {\rho _{\text{c}}}\varepsilon \left( {{j_{{\text{slip}}}} \cdot \nabla } \right)j =- \\& \nabla p - \nabla \cdot {\tau _{{\text{Gm}}}} + \rho g{{ - }}\nabla \cdot \left[ {{\rho _{\text{c}}}\left( {1 + {\phi _{\text{c}}}\varepsilon } \right){u_{{\text{slip}}}}{j_{{\text{slip}}}}^T} \right] - \\& {\rho _{\text{c}}}\varepsilon \left[ {\left( {j \cdot \nabla } \right){j_{{\text{slip}}}} + \left( {\nabla \cdot \left( {{D_{{\text{md}}}}\nabla {\phi _{\text{d}}}} \right)} \right)j} \right] \end{split} $$ (1) 式中：ρ为混合物密度，kg/m3；j为混合物体积平均速度，m/s；ρc为连续相密度，kg/m3；$\nabla $为梯度运算；ε为混合物中两相密度差的减小量；jslip为滑动通量，m/s；p为混合物压力，MPa；τGm为混合物粘性和湍流应力之和；uslip为滑动速度，m/s；Dmd为湍流扩散系数，m2/s；g为重力加速度，m/s2；ϕc和ϕd分别为携砂液中连续相和分散相的体积分数，无量纲。
混合物密度表示为
$$ \rho = {\phi _{\text{c}}}{\rho _{\text{c}}} + {\phi _{\text{d}}}{\rho _{\text{d}}} $$ (2) 式中：ρd为分散相密度，kg/m3。
混合物体积平均速度为
$$ j = {\phi _{\text{d}}}{u_{\text{d}}} + {\phi _{\text{c}}}{u_{\text{c}}} $$ (3) 式中：uc和ud为连续相速度矢量和分散相速度矢量。
混合物中两相密度差的减小量为
$$ \varepsilon = \frac{{{\rho _{\text{d}}} - {\rho _{\text{c}}}}}{{{\rho _{\text{c}}}}} $$ (4) 滑动通量为
$$ {j_{{\text{slip}}}} = {\phi _{\text{d}}}{\phi _{\text{c}}}{u_{{\text{slip}}}} $$ (5) 式中：uslip为示两相之间的相对速度，m/s。
混合物黏性和湍流应力之和为
$$ \begin{gathered} {\tau _{{\text{Gm}}}} = \left( {\mu + {\mu _{\text{T}}}} \right)\left[ {\nabla j + \nabla {j^T}} \right] \\ - \frac{2}{3}\left( {\mu + {\mu _{\text{T}}}} \right)\left( {\nabla \cdot j} \right){\text{I }}-\frac{{\text{2}}}{{\text{3}}}\rho k{\text{I}} \\ \end{gathered} $$ (6) 式中：μ为混合物黏度，Pa·s；μT为湍流黏度，Pa·s；k为可用的湍流动能，m2/s2。
混合物湍流扩散系数为
$$ {D_{{\text{md}}}} = \frac{{{\mu _{\text{T}}}}}{{\rho {\sigma _{\text{T}}}}} $$ (7) 式中：σT为湍流粒子施密特数，无量纲。
$$ \frac{{\partial \rho }}{{\partial t}} + \nabla \cdot \left( {\rho u} \right) = 0 $$ (8) 固相输送方程为
$$ \frac{\partial }{{\partial t}}\left( {{\phi _{\text{d}}}{\rho _{\text{d}}}} \right) + \nabla \cdot \left( {{\phi _{\text{d}}}{\rho _{\text{d}}}{u_{\text{d}}}} \right) = \nabla \cdot \left( {{\rho _{\text{d}}}{D_{{\text{md}}}}\nabla {\phi _{\text{d}}}} \right) $$ (9) 滑移速度为
$$ \begin{gathered} \frac{3}{4}\frac{{{C_{\text{d}}}}}{{{d_{\text{d}}}}}\left| {{u_{{\text{slip}}}}} \right|{u_{{\text{slip}}}} = - \frac{{\left( {\rho {{ - }}{\rho _{\text{d}}}} \right)}}{{{\rho _{\text{c}}}}}\left( { - \frac{{\partial j}}{{\partial t}} - \left( {j \cdot \nabla } \right)j + g} \right) \\ \end{gathered} $$ (10) 式中：Cd为颗粒阻力系数，无量纲，计算方式[28]如下：
$$ {C_{\text{d}}} = \frac{{24}}{{{{R}}{{{e}}_{\text{p}}}}}\left( {1 + A{{Re}}_{\text{p}}^B} \right) + \frac{C}{{1 + D/{{R}}{{{e}}_{\text{p}}}}} $$ (11) 式中：Rep为颗粒雷诺数；A、B、C和D为经验系数。
$$ 0 \lt {S_{\text{p}}} = \frac{{{A_{{\text{sphere}}}}}}{{{A_{{\text{particle}}}}}} \leqslant 1 $$ (12) $$ \begin{gathered} A = {{\mathrm{e}}^{2.328\;8 - 6.458\;1{S_{\text{p}}} + 2.448\;6S_{\text{p}}^2}} \\ B = 0.096\;4 + 0.556\;5{S_{\text{p}}} \\ C = {{\mathrm{e}}^{4.905 - 13.894\;4{S_{\text{p}}} + 18.422\;2S_{\text{p}}^2 - 10.259\;9S_{\text{p}}^3}} \\ D = {{\mathrm{e}}^{1.468\;1 + 12.258\;4{S_{\text{p}}} - 20.732\;2S_{\text{p}}^2 + 15.885\;5_{\text{p}}^3}} \\ \end{gathered} $$ (13) 式中：e为自然常数；Sp为支撑剂颗粒球度。
3. 数值模拟结果
与上述数学模型类似的Mixture模型同样被应用于其他关于支撑剂输送模拟的研究中，且与实验结果对比展示了良好的准确性[29-31]。因此，在借助上述缝网模型和Mixture模型，开展了不同携砂液黏度、排量和支撑剂粒径条件下，联通缝网和未联通缝网内支撑剂分布特征的对比分析，模拟参数见表2。
表 2 数值模拟中所使用的参数Table 2. Parameters used in the simulation参数 数值 支撑剂粒径/目(mm) 50/80/100/120(0.3/0.18/0.15/0.125) 支撑剂绝对密度/(kg·m−3) 2 200 支撑剂球度 0.9 携砂液黏度/(mPa·s) 1/3/5/7 携砂液密度/(kg·m−3) 1 000 携砂液排量/(m3·min−1) 8/10/12/14 携砂液砂比/% 15 3.1 携砂液黏度影响
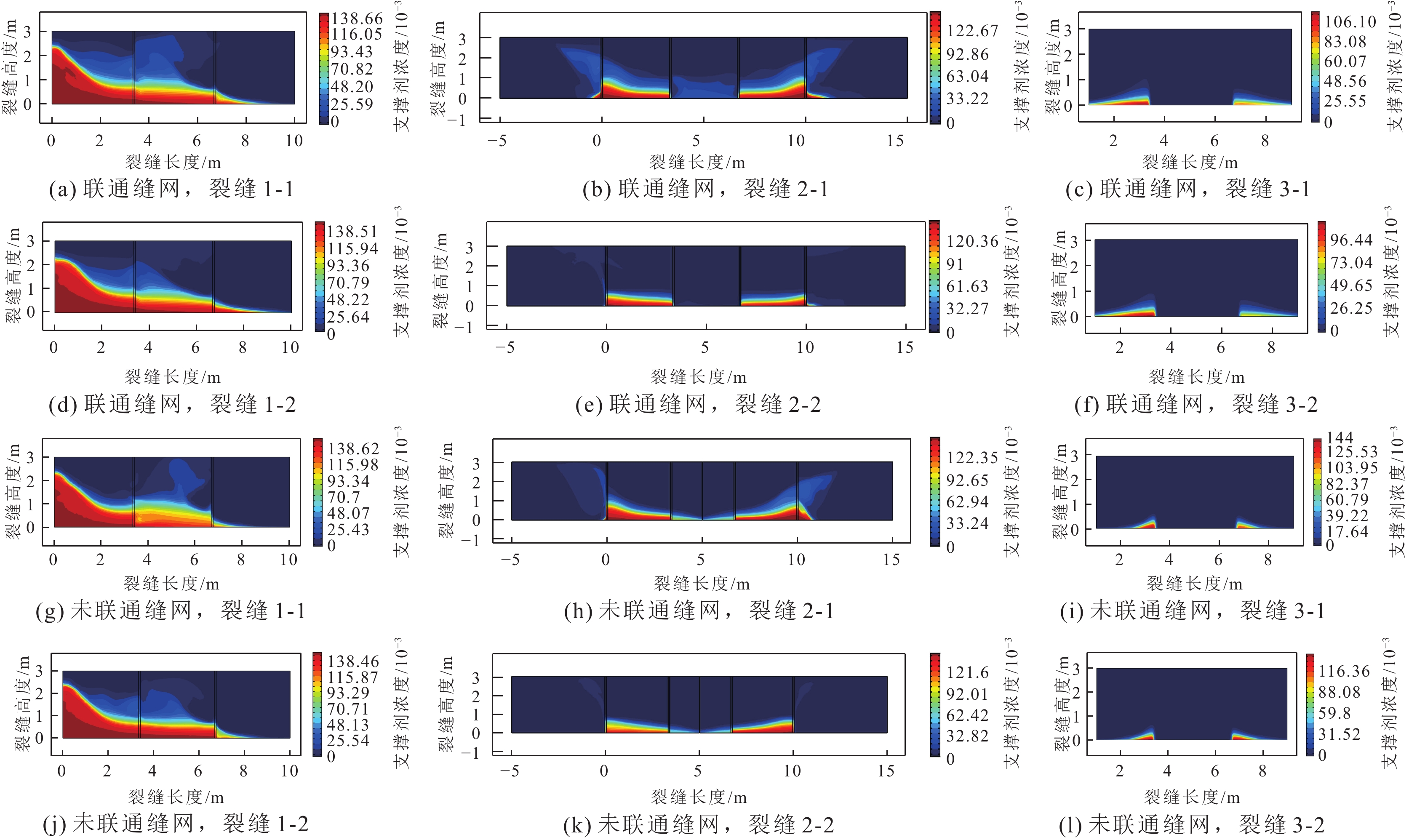
对于联通缝网和未联通缝网中各级裂缝内支撑剂的分布特征，以携砂液黏度为1 mPa·s时的模拟结果为例进行说明，如图3所示。可以看出，支撑剂主要铺置在缝网主裂缝内，二级裂缝和三级裂缝中填砂较少。两种形态的缝网相比，联通缝网中主裂缝内支撑剂的输送距离更长，且二级裂缝的分流对其主裂缝内支撑剂的铺砂浓度和高度影响较小。但是，联通缝网内两簇裂缝中携砂液在二级裂缝中流动互相干扰，使得二级裂缝的中间部分几乎没有支撑剂分布，而未联通缝网的二级裂缝彼此没有干扰，其二级裂缝中间部分有少量支撑剂分布。此外，联通缝网的三级裂缝内支撑剂的输送距离和铺置范围均优于未联通缝网，但是其最大铺砂浓度较未联通缝网小。
![]()
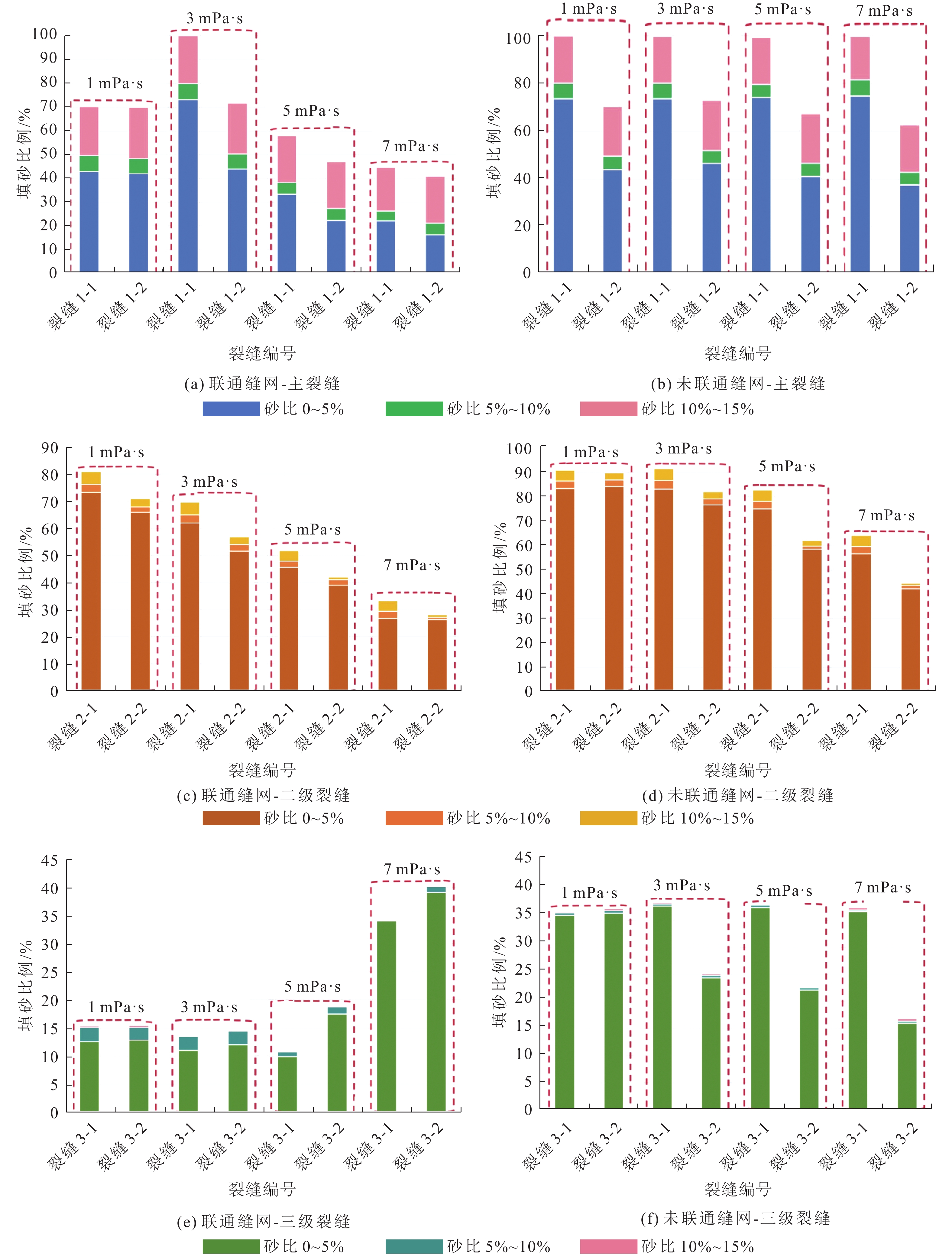
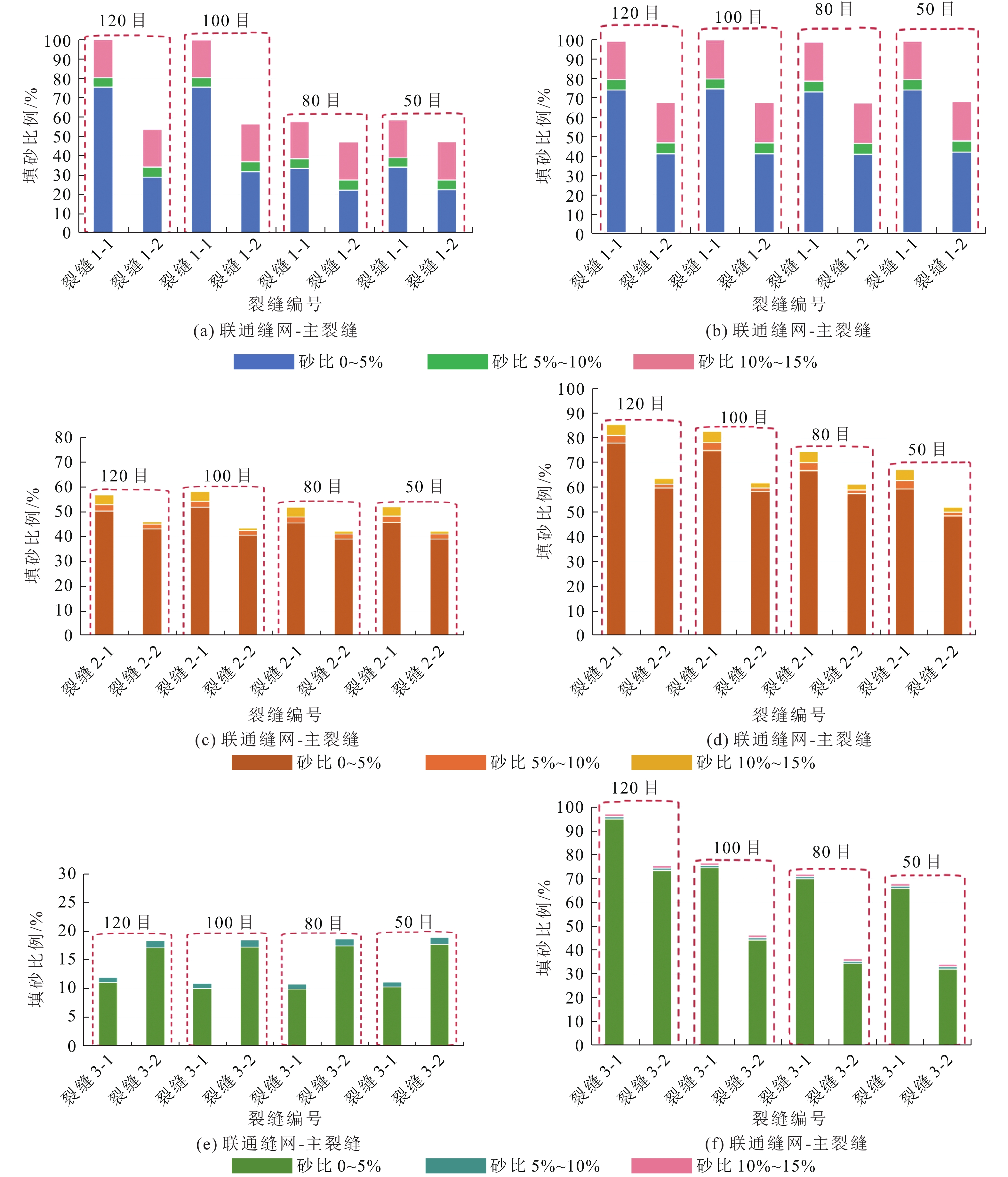
图 3 携砂液黏度为1 mPa·s时联通和未联通缝网各级裂缝内支撑剂分布对比Figure 3. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with slurry viscosity of 1 mPa·s为了进一步量化说明支撑剂在两种类型缝网中的分布情况，按照携砂液入口的砂比，将其均分为3个区间，即0%～5%的低浓度区域，5%～10%的中浓度区域和10%～15%的高浓度区域，对不同黏度条件下各级裂缝内支撑剂的填砂比例进行分析，如图4所示，这里的填砂比例指的是某级别裂缝中铺砂浓度大于0%的区域体积占该级别裂缝总体积的比例。模拟结果显示，无论是联通缝网还是非联通缝网，低浓度区域在各级裂缝中均占最大比例，特别是未联通缝网的这一特征由主裂缝到二级裂缝再到三级裂缝愈发明显。同时，随着携砂液黏度的增大，未联通缝网中各级裂缝的填砂比例逐渐降低，特别是裂缝2-1和裂缝2-2的填砂比例由携砂液黏度为1 mPa·s时的90.78%和89.72%，下降至携砂液黏度为7 mPa·s时的64.08%和44.41%。相较而言，联通缝网中两条主裂缝填砂比例在携砂液黏度为3 mPa·s时达到最大，分别为99.95%和71.58%，而三级裂缝中的填砂比例随着携砂液黏度的提高而不断增大，当携砂液黏度为7 mPa·s时，两条三级裂缝的填砂比例分别达到34.18%和40.10%。
![]()
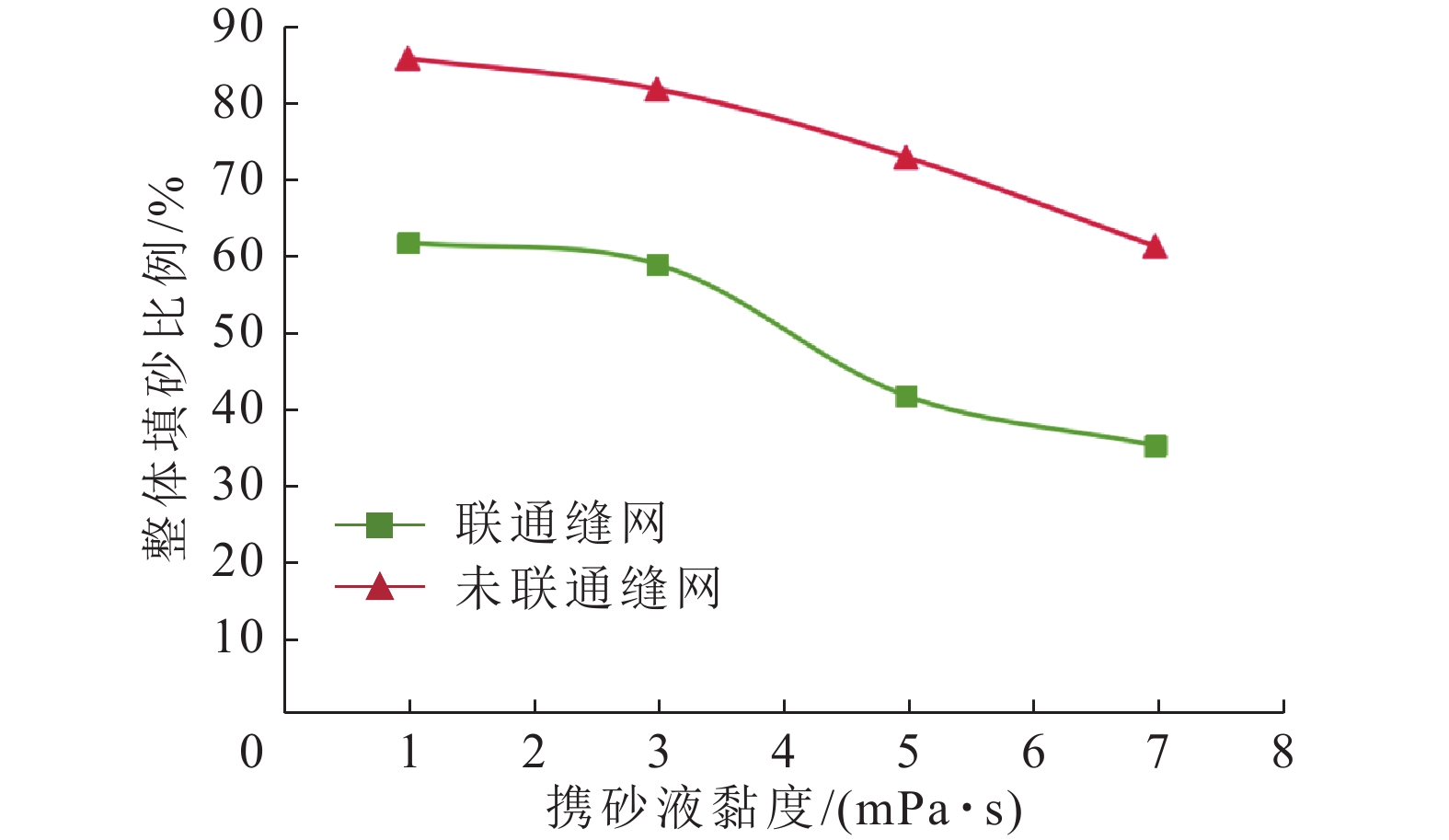
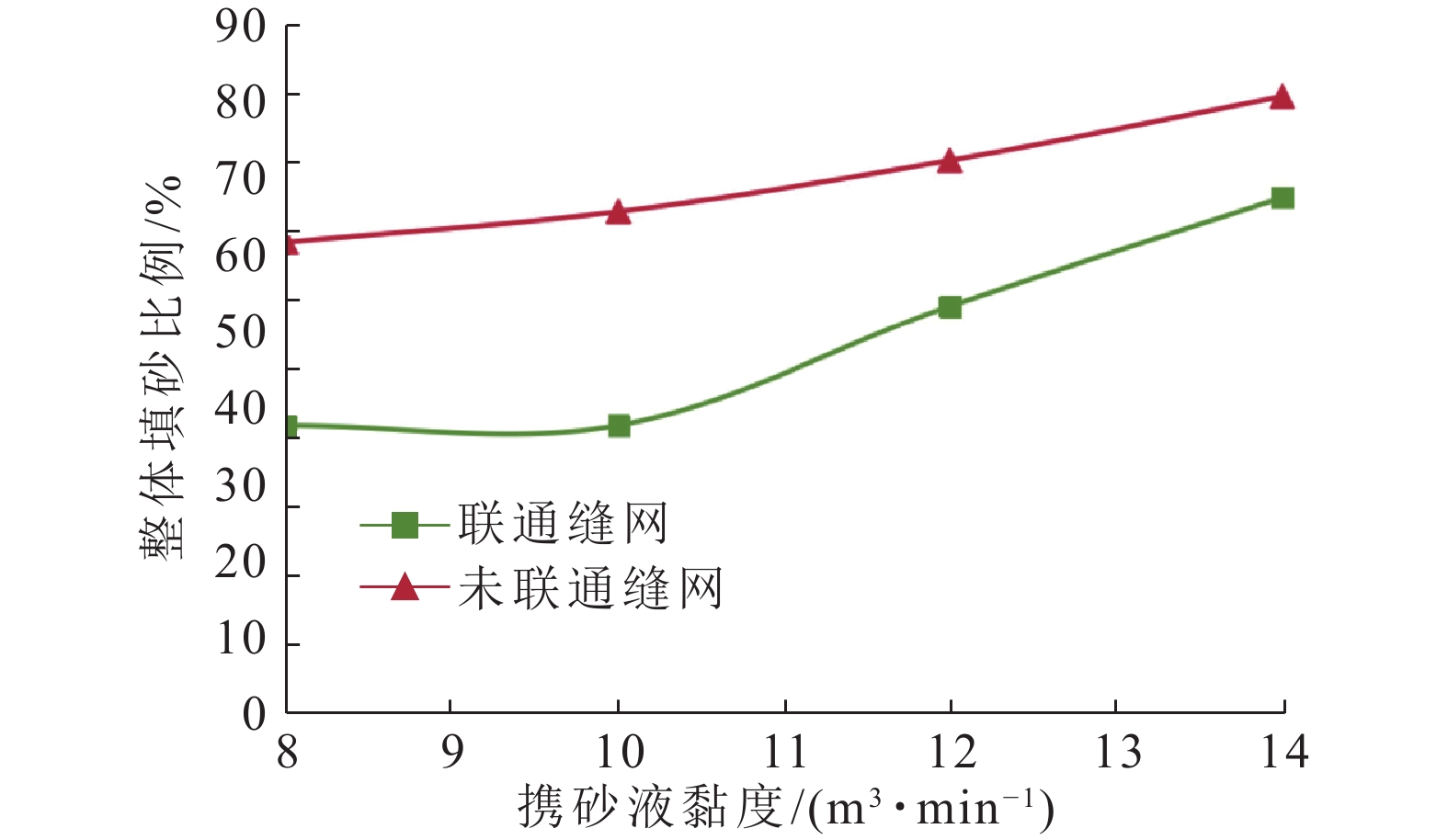
图 4 不同黏度条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例Figure 4. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different slurry viscosity就缝网整体填砂比例而言，联通缝网和未联通缝网之间的差别如图5所示。显然，两种缝网整体的填砂比例均随着携砂液黏度的提高而减小，特别是携砂液黏度由3 mPa·s提高到5 mPa·s时联通缝网整体的填砂比例显著下降。而且未联通缝网的整体填砂比例均高于联通缝网，4种携砂液黏度条件下，未联通缝网的整体填砂比例比联通缝网的整体填砂比例平均高出56.60%。
![]()
图 5 不同黏度条件下联通裂缝和未联通缝网整体填砂比例Figure 5. Total sand filling ratio of connected and unconnected fracture networks under different slurry viscosity3.2 携砂液排量影响
携砂液排量对两种缝网内支撑剂铺置情况的影响以携砂液排量为14 m3/min时的模拟结果为例进行说明，如图6所示，其分布特征与图3中支撑剂铺置情况类似（该结果的携砂液排量为10 m3/min）。不同的是，携砂液排量提高后，主裂缝和二级裂缝内支撑剂的铺砂高度和输送距离均得到提高。
![]()
图 6 携砂液排量为14 m3/min时联通和未联通缝网各级裂缝内支撑剂分布对比Figure 6. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with slurry displacement of 14 m3/min图7为不同携砂液排量条件下支撑剂在两种形态缝网中不同铺砂浓度的分布情况。模拟结果表明，低浓度铺砂区域仍然在各级裂缝中占据较大面积，但是随着携砂液排量的提高，各级裂缝中总体的填砂比例不断增大。而且相较于未联通缝网，提高携砂液排量对未联通缝网的影响更加显著，特别是未联通缝网中裂缝3-1的填砂比例由携砂液排量为8 m3/min时的11.35%增加到携砂液排量为14 m3/min时的98.77%。此外，虽然各级裂缝中靠近携砂液入口的裂缝填砂比例更高，但是随着携砂液排量的增大，两者的差距在逐渐减小。
![]()
图 7 不同排量条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例Figure 7. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different slurry displacement不同排量条件下联通裂缝和未联通缝网的整体填砂比例如图8所示。可以看出，随着携砂液排量的增大，未联通缝网的整体填砂比例基本呈线性增大，而联通缝网的整体填砂比例在携砂液排量增大到10 m3/min后提高更加显著。此外，4种携砂液排量条件下，未联通缝网的整体填砂比例比联通缝网的整体填砂比例平均高出48.55%。
![]()
图 8 不同排量条件下联通裂缝和未联通缝网整体填砂比例Figure 8. Total sand filling ratio of connected and unconnected fracture networks under different slurry displacement3.3 支撑剂粒径影响
以支撑剂粒径为120目时缝网内支撑剂的模拟结果为例，展示支撑剂粒径对两种缝网内支撑剂铺置情况的影响，如图9所示，其分布特征同样与图3中支撑剂铺置情况类似（该结果的支撑剂粒径为50目）。不同的是，携砂液排量提高后，主裂缝内支撑剂的输送距离得到延长。
![]()
图 9 支撑剂粒径为120目时联通和未联通缝网各级裂缝内支撑剂分布对比Figure 9. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with a proppant particle size of 120 mesh支撑剂粒径变化对两种缝网中各级裂缝内支撑剂分布情况的影响如图10所示。对于未联通缝网，增大支撑剂粒径对主裂缝内支撑剂铺置情况的影响较小，但是显著减小了二级裂缝和三级裂缝内支撑剂的填砂比例。120目支撑剂在裂缝2-1和裂缝2-2中的填砂比例分别为85.48%和63.6%，采用50目支撑剂后其填砂比例分别减小至67.29%和52.34%。同样的，120目支撑剂在裂缝3-1和裂缝3-2中的填砂比例分别为96.37%和74.72%，采用50目支撑剂后其填砂比例分别减小至67.32%和33.31%。而对联通缝网而言，增大支撑剂粒径主要影响了主裂缝内支撑剂的分布，裂缝1-1和裂缝1-2内的填砂比例分别由支撑剂120目时的99.8%和53.9%，减小至支撑剂50目时的58.64%和47.28%。
![]()
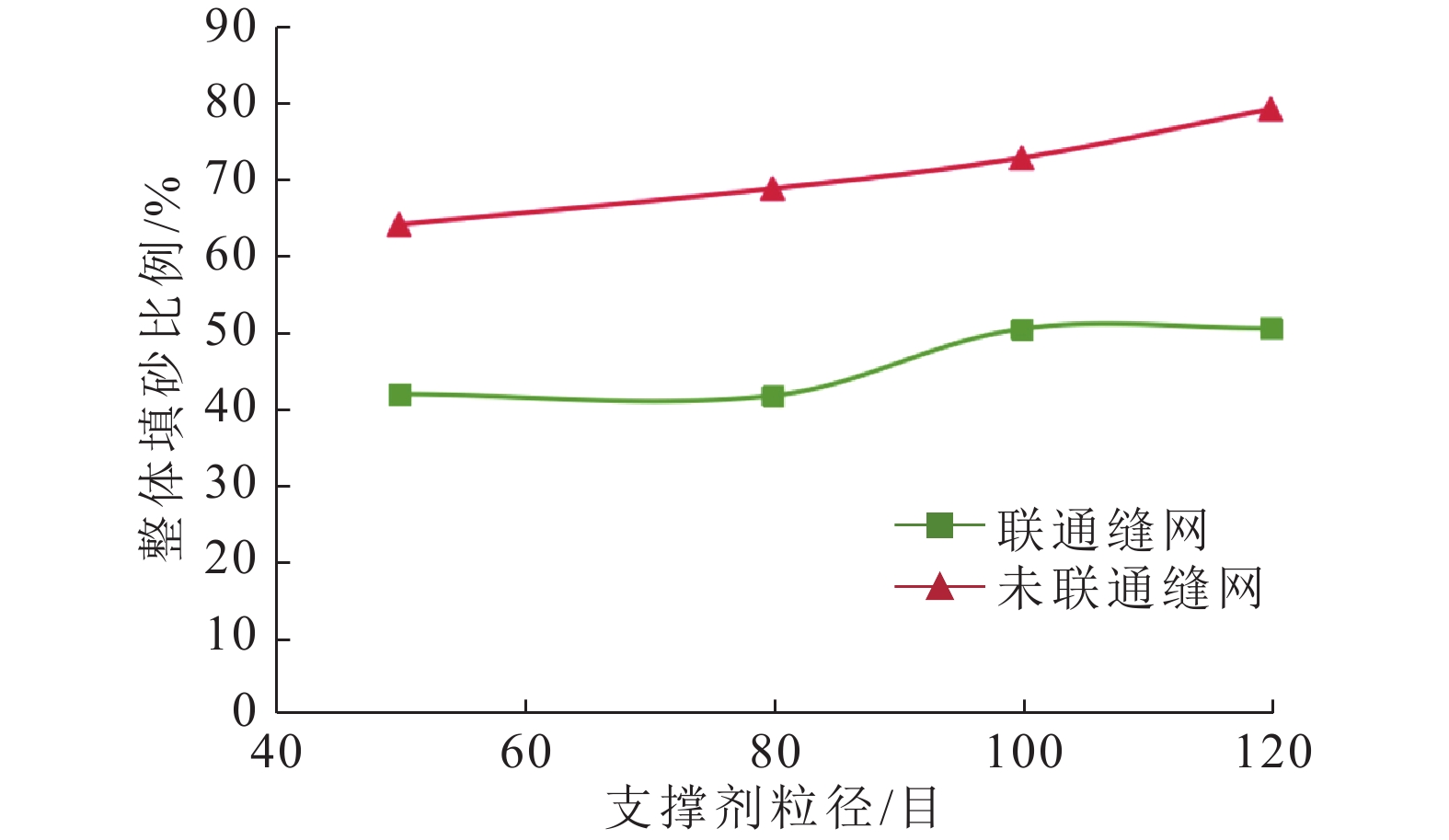
图 10 不同粒径条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例Figure 10. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different proppant diameters支撑剂粒径对裂缝网络内整体填砂比例的影响如图11所示。可以看出，随着支撑剂粒径的增大，两种缝网内整体填砂比例均在不断减小，且支撑剂粒径由100目增大至80目时，联通缝网内整体填砂比例显著降低。4种支撑剂粒径条件下，未联通缝网的整体填砂比例比联通缝网的整体填砂比例平均高出54.75%。
![]()
图 11 不同排量条件下联通裂缝和未联通缝网整体填砂比例Figure 11. Total sand filling ratio of connected and unconnected fracture networks under different proppant diameters4. 结 论
1）对于多簇相互联通的裂缝网络，由于携砂液在缝网联通部分的流动互相干扰，该部分几乎没有支撑剂分布，但是支撑剂在联通缝网的主裂缝内输送距离更远。
2）对裂缝网络整体的填砂比例而言，未联通缝网的整体填砂比例要远高于联通缝网，在本研究的模拟参数条件下平均高出53.3%。
3）两种类型缝网中各级裂缝内铺砂浓度小于5%的区域均占较大比例，同时降低携砂液黏度、提高携砂液排量、使用粒径较小的支撑剂均可以有效改善两种缝网中支撑剂的整体填砂比例，特别是联通缝网中携砂液排量应不小于12 m3/min，支撑剂粒径不大于100目。
4）与以往研究相比，本研究既体现了水平井筒对多簇裂缝的串联，也考虑了各簇缝网内部的联通，揭示了煤层气压裂开发过程中多簇联通和未联通缝网内支撑剂分布的差异，相关结论可以为割理发育程度不同的煤层压裂施工中的携砂液黏度、排量和支撑剂粒径优化设计提供必要的技术支撑。
-
![]()
图 1 煤层水力压裂后复杂缝网示意
Figure 1. Schematic diagram of complex fracture networks after hydraulic fracturing in coal seam
![]()
图 2 数值模拟中缝网几何模型
Figure 2. Geometric model of fracture networks in numerical simulation
![]()
图 3 携砂液黏度为1 mPa·s时联通和未联通缝网各级裂缝内支撑剂分布对比
Figure 3. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with slurry viscosity of 1 mPa·s
![]()
图 4 不同黏度条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例
Figure 4. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different slurry viscosity
![]()
图 5 不同黏度条件下联通裂缝和未联通缝网整体填砂比例
Figure 5. Total sand filling ratio of connected and unconnected fracture networks under different slurry viscosity
![]()
图 6 携砂液排量为14 m3/min时联通和未联通缝网各级裂缝内支撑剂分布对比
Figure 6. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with slurry displacement of 14 m3/min
![]()
图 7 不同排量条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例
Figure 7. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different slurry displacement
![]()
图 8 不同排量条件下联通裂缝和未联通缝网整体填砂比例
Figure 8. Total sand filling ratio of connected and unconnected fracture networks under different slurry displacement
![]()
图 9 支撑剂粒径为120目时联通和未联通缝网各级裂缝内支撑剂分布对比
Figure 9. Comparison of proppant distribution in different levels of fractures in connected and unconnected fracture networks with a proppant particle size of 120 mesh
![]()
图 10 不同粒径条件下联通裂缝和未联通缝网中各级裂缝内的填砂比例
Figure 10. Sand filling ratio in different levels of fractures in connected and unconnected fracture networks under different proppant diameters
![]()
图 11 不同排量条件下联通裂缝和未联通缝网整体填砂比例
Figure 11. Total sand filling ratio of connected and unconnected fracture networks under different proppant diameters
表 1 缝网几何参数
Table 1 Geometric parameters of fracture networks
参数 数值 主裂缝长度/m 10 三级裂缝长度/m 8 裂缝宽度/cm 1 水平井筒长度/m 20 簇间距/m 10 三级裂缝间距/m 3.33 联通二级裂缝长度/m 20 未联通二级裂缝长度/m 9.995 裂缝高度/m 3 水平井筒半径/cm 7 二级裂缝间距/m 3.33 未联通缝网簇间二级裂缝间距/m 0.01 
下载: 导出CSV
表 2 数值模拟中所使用的参数
Table 2 Parameters used in the simulation
参数 数值 支撑剂粒径/目(mm) 50/80/100/120(0.3/0.18/0.15/0.125) 支撑剂绝对密度/(kg·m−3) 2 200 支撑剂球度 0.9 携砂液黏度/(mPa·s) 1/3/5/7 携砂液密度/(kg·m−3) 1 000 携砂液排量/(m3·min−1) 8/10/12/14 携砂液砂比/% 15
下载: 导出CSV
-
[1] 孙东玲,梁运培,黄旭超,等. 新疆大倾角多煤组煤矿区煤层气开发利用进展与前景[J]. 煤炭科学技术,2023,51(S1):162−172. SUN Dongling,LIANG Yunpei,HUANG Xuchao,et al. Progress and prospects of coalbedmethane developmentand utilization in coal mining areas with large dip angle and multiplecoal groups in Xinjiang[J]. Coal Science andTechnology,2023,51(S1):162−172.
[2] 徐凤银,肖芝华,陈东,等. 我国煤层气开发技术现状与发展方向[J]. 煤炭科学技术,2019,47(10):205−215. XU Fengyin,XIAO Zhihua,CHEN Dong,et al. Current status and development direction of coalbed methane exploration technology in China[J]. Coal Science and Technology,2019,47(10):205−215.
[3] 姚红生,杨松,刘晓,等. 低效煤层气井多次压裂增效开发技术研究[J]. 煤炭科学技术,2022,50(9):121−129. YAO Hongsheng,YANG Song,LIU Xiao,et al. Research on efficiency-enhancing development technology of multiple fracturing in low-efficiency CBM wells[J]. Coal Science and Technology,2022,50(9):121−129.
[4] 申鹏磊,吕帅锋,李贵山,等. 深部煤层气水平井水力压裂技术:以沁水盆地长治北地区为例[J]. 煤炭学报,2021,46(8):2488−2500. SHEN Penglei,LYU Shuaifeng,LI Guishan,et al. Hydraulic fracturing technology for deep coalbed methane horizontal wells:A case study in North Changzhi area of Qinshui Basin[J]. Journal of China Coal Society,2021,46(8):2488−2500.
[5] 丛日超,王海柱,李根生,等. 超临界CO2聚能压裂开发煤层气可行性研究[J]. 煤炭学报,2023,48(8):3162−3171. CONG Richao,WANG Haizhu,LI Gensheng,et al. Feasibility on exploitation of coalbed methane by SC-CO2 shock fracturing[J]. Journal of China Coal Society,2023,48(8):3162−3171.
[6] 陈捷,胡海洋,刘立,等. 薄煤层水力裂缝宽度变化对支撑剂运移的影响[J]. 断块油气田,2023,30(5):728−733,750. CHEN Jie,HU Haiyang,LIU Li,et al. The influence of hydraulic fracture width change on proppant transport in thin coal seam[J]. Fault-Block Oil & Gas Field,2023,30(5):728−733,750.
[7] 苏现波,范渐,王然,等. 煤储层水力压裂裂缝内支撑剂运移控制因素实验研究[J]. 煤田地质与勘探,2023,51(6):62−73. SU Xianbo,FAN Jian,WANG Ran,et al. An experimental study on factors controlling the proppant transport in hydraulic fractures of coal reservoirs[J]. Coal Geology & Exploration,2023,51(6):62−73.
[8] 王生维,熊章凯,吕帅锋,等. 煤储层水力压裂裂缝中支撑剂特征及研究意义[J]. 煤田地质与勘探,2022,50(3):137−145. doi: 10.12363/issn.1001-1986.21.12.0813 WANG Shengwei,XIONG Zhangkai,LYU Shuaifeng,et al. Characteristics and significance of proppant in hydraulic fractures in coal reservoirs[J]. Coal Geology & Exploration,2022,50(3):137−145. doi: 10.12363/issn.1001-1986.21.12.0813
[9] 张苗,邹明俊,吕乐乐,等. 水力压裂过程中陶粒支撑剂运移规律及粒级配比优化[J]. 煤矿安全,2022,53(2):16−19,26. ZHANG Miao,ZOU Mingjun,LYU Lele,et al. Migration law of ceramsite proppant and its granularity matching during coalbed methane fracturing[J]. Safety in Coal Mines,2022,53(2):16−19,26.
[10] LI H Z,HUANG B X,ZHAO X L,et al. Experimental investigation on proppant transport and distribution characteristics in coal hydraulic fractures under true triaxial stresses[J]. Journal of Petroleum Science and Engineering,2022,218:110993. doi: 10.1016/j.petrol.2022.110993
[11] LAMEI RAMANDI H,PIRZADA M A,SAYDAM S,et al. Digital and experimental rock analysis of proppant injection into naturally fractured coal[J]. Fuel,2021,286:119368. doi: 10.1016/j.fuel.2020.119368
[12] 郭天魁,宫远志,刘晓强,等. 复杂裂缝中支撑剂运移铺置规律数值模拟[J]. 中国石油大学学报(自然科学版),2022,46(3):89−95. GUO Tiankui,GONG Yuanzhi,LIU Xiaoqiang,et al. Numerical simulation of proppant migration and distribution in complex fractures[J]. Journal of China University of Petroleum (Edition of Natural Science),2022,46(3):89−95.
[13] 张艳博,徐加祥,刘哲,等. 二级裂缝倾角对复杂缝网中支撑剂输送的影响[J]. 大庆石油地质与开发,2023,42(1):91-99. ZHANG Yanbo,XU Jiaxiang ,LIU Zhe,et al. Effect of secondary fracture dip on proppant transport in complex fracture network[J]. Petroleum Geology & Oilfield Development in Daqing,2023,42(1):91-99.
[14] XU J X,GAO R,YANG L F,et al. A 3D tortuous fracture network construction approach to analyze proppant distribution in post-fractured shale[J]. Journal of Petroleum Science and Engineering,2022,208:109676. doi: 10.1016/j.petrol.2021.109676
[15] MAO S W,ZHANG Z,CHUN T S,et al. Field-scale numerical investigation of proppant transport among multicluster hydraulic fractures[J]. SPE Journal,2021,26(1):307−323.
[16] 杨鹏,张士诚,邹雨时,等. 井平台多井压裂裂缝扩展、支撑剂运移规律与参数优化[J]. 石油勘探与开发,2023,50(5):1065−1073. YANG Peng,ZHANG Shicheng,ZOU Yushi,et al. Fracture propagation,proppant transport and parameter optimization of multi-well pad fracturing treatment[J]. Petroleum Exploration and Development,2023,50(5):1065−1073.
[17] 安琦,杨帆,杨睿月,等. 鄂尔多斯盆地神府区块深部煤层气体积压裂实践与认识[J]. 煤炭学报,2024,49(5):2376−2393. AN Qi,YANG Fan,YANG Ruiyue,et al. Practice and understanding of deep coalbed methane massive hydraulic fracturing in shenfu block,ordos basin[J]. Journal of China Coal Society,2024,49(5):2376−2393.
[18] 贺艳祥,徐强,张争光,等. 沁水盆地西南缘煤层气水力压裂裂缝分布特征及主控因素研究[J]. 煤炭工程,2023,55(S1):141−147. HE Yanxiang,XU Xiang,ZHANG Zhengguang,et al. Distribution characteristics and main controlling factors of hydraulic fracturing fractures of coalbed methane in the southwestern margin of Qinshui Basin[J]. Coal Engineering,2023,55(S1):141−147.
[19] 李倩,李童,蔡益栋,等. 煤层气储层水力裂缝扩展特征与控因研究进展[J]. 煤炭学报,2023,48(12):4443−4460. LI Qian,LI Tong,CAI Yidong,et al. Research progress on hydraulic fracture characteristics and controlling factors of coalbed methane reservoirs[J]. Journal of China Coal Society,2023,48(12):4443−4460.
[20] 周大庆,陈雅楠,陈会向,等. 基于VOF模型的深隧系统入流竖井两相流模拟[J]. 中国给水排水,2023,39(19):132−138. ZHOU Daqing,CHEN Yanan,CHEN Huixiang,et al. Simulation of two-phase flow in inflow shaft of deep tunnel drainage system based on VOF model[J]. CHINA WATER & WASTEWATER,2023,39(19):132−138.
[21] 钟安海,郭天魁. 水平井多簇支撑剂分布数值模拟[J]. 深圳大学学报(理工版),2022,39(5):576−583. doi: 10.3724/SP.J.1249.2022.05576 ZHONG Anhai,GUO Tiankui. Numerical simulation of multi-cluster proppant distribution in horizontal wells[J]. Journal of Shenzhen University (Science and Engineering),2022,39(5):576−583. doi: 10.3724/SP.J.1249.2022.05576
[22] 左伟芹,谢坤容,韩红凯,等. 清洁压裂液携带作用下支撑剂运移铺置规律研究[J]. 煤矿安全,2022,53(12):42−47. ZUO Weiqin,XIE Kunrong,HAN Hongkai,et al. Study on proppant migration and placement under sand-carrying action of clean fracturing fluid[J]. Safety in Coal Mines,2022,53(12):42−47.
[23] 王猛,王海柱,李根生,等. 超临界CO2压裂缝内携砂数值模拟[J]. 石油机械,2018,46(11):72−78,84. WANG Meng,WANG Haizhu,LI Gensheng,et al. Numerical study of proppant transport with supercritical CO2 in fracture[J]. China Petroleum Machinery,2018,46(11):72−78,84.
[24] 张涛,郭建春,刘伟. 清水压裂中支撑剂输送沉降行为的CFD模拟[J]. 西南石油大学学报(自然科学版),2014,36(1):74−82. ZHANG Tao,GUO Jianchun,LIU Wei. CFD simulation of proppant transportation and settling in water fracture treatments[J]. Journal of Southwest Petroleum University (Science & Technology Edition),2014,36(1):74−82.
[25] 刘春亭,李明忠,郝丽华,等. 水力裂缝内支撑剂输送沉降行为数值仿真[J]. 大庆石油地质与开发,2018,37(5):86−93. LIU Chunting,LI Mingzhong,HAO Lihua,et al. Numerical simulation of the proppant transportation and setting in the hydraulic fracture[J]. Petroleum Geology & Oilfield Development in Daqing,2018,37(5):86−93.
[26] 任岚,林辰,林然,等. 复杂裂缝中低密度支撑剂铺置数值模拟[J]. 大庆石油地质与开发,2021,40(6):52−61. REN Lan,LIN Chen,LIN Ran,et al. Numerical simulation of the low-density proppant placement in complex fractures[J]. Petroleum Geology & Oilfield Development in Daqing,2021,40(6):52−61.
[27] 林啸,杨兆中,胡月,等. 页岩体积压裂支撑剂铺置运移模拟及其应用[J]. 大庆石油地质与开发,2021,40(6):151−157. LIN Xiao,YANG Zhaozhong,HU Yue,et al. Simulation of proppant placement and migration in shale volume fracturing and its application[J]. Petroleum Geology & Oilfield Development in Daqing,2021,40(6):151−157.
[28] 徐加祥,丁云宏,杨立峰,等. 压裂支撑剂在迂曲微裂缝中输送与分布规律[J]. 石油学报,2019,40(8):965−974. doi: 10.7623/syxb201908007 XU Jiaxiang,DING Yunhong,YANG Lifeng,et al. Transportation and distribution laws of proppants in tortuous micro-fractures[J]. Acta Petrolei Sinica,2019,40(8):965−974. doi: 10.7623/syxb201908007
[29] YUE M,ZHANG Q T,ZHU W Y,et al. Effects of proppant distribution in fracture networks on horizontal well performance[J]. Journal of Petroleum Science and Engineering,2020,187:106816. doi: 10.1016/j.petrol.2019.106816
[30] RIVAS E,GRACIE R. Numerical considerations for the simulation of proppant transport through fractures[J]. Journal of Petroleum Science and Engineering,2019,180:1006−1021. doi: 10.1016/j.petrol.2019.05.064
[31] 张涛, 蹇胤霖, 何乐, 等. 压裂复杂裂缝中支撑剂输送数值模拟研究[J]. 油气地质与采收率,2024,31(3):123−136. ZHANG Tao, JIAN Yinlin, HE Le, et al. Numerical simulation study of proppant transport in complex hydraulic fractures[J]. Petroleum Geology and Recovery Efficiency,2024,31(3):123−136.
计量
- 文章访问数: 17
- HTML全文浏览量: 5
- PDF下载量: 29
